Equilibrium and out of equilibrium statistical mechanics of geophysical flows
In many applications of fluid dynamics, one of the most important problem is the prediction of the very high Reynolds large-scale flows. The highly turbulent nature of such flows, for instance ocean circulation or atmosphere, renders a probabilistic description desirable. We describe theoretical progresses in order to use statistical mechanics ideas for these problems and some applications to real geophysical flows.
Review on the equilibrium
statistical mechanics of geophysical flows:
F.
Bouchet and A. Venaille, Physics Reports, 2009.
Applications of Stat. Mech. to real geophysical
flows
Jupiter-Equilibrium

The
Jupiter's Great Red Spot
An
equilibrium statistical mechanics explanation of the
self-organization of geophysical flows has been proposed
by Robert-Sommeria and Miller (RSM). The RSM theory has
been successfully applied to the Jupiterís troposphere:
cyclones, anticyclones and jets have been quantitatively
described by this theory
(see
the poster A
statistical equilibrium model of the Great Red Spot and
other Jovian vortices and the publications Bouchet-Sommeria-2002
and Bouchet-Dumont-2002)
Ocean-Equilibrium

The see
surface height of the North Atlantic.
A
natural question is to know if some aspects of the large
scale organization of ocean dynamics, the map of currents
for instance, can be understood in the context of
equilibrium statistical mechanics.
Ocean rings are the most common vortices in oceans. They
explain most of the kinetic energy variability of ocean
dynamics. We have explained the structures, shapes, and
velocity fields of ocean rings using equilibrium
statistical mechanics. We have also explained from
statistical mechanics why the complex mixing resulting
from turbulence leads to the formation of strong mid-basin
jets like the Gulf Stream in the Atlantic Ocean and the
Kuroshio in the Pacific Ocean.
(see
the poster Equilibrium
Statistical Mechanics of Mid Basin Eastward Jets and of
Ocean Vortices and the publications Bouchet--Venaille--JPO--2011
and F.
Bouchet and A. Venaille, Physics Reports, 2009.)
Theoretical progresses in the Stat. Mech. of 2D and geophysical flows
Out of equilibrium phase transitions
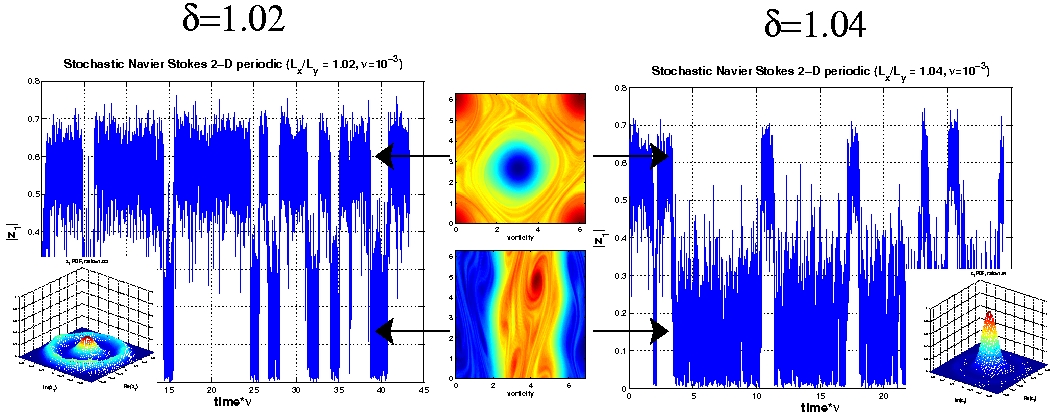
Prediction
of the bistability of turbulent flows for the 2D Navier
Stokes Equations
(please
see the poster Out
of Equilibrium Phase Transitions in 2D and Geophysical
Flows and the publications Bouchet-Simonnet-PRL-2009
and F.
Bouchet and A. Venaille, Physics Reports, 2009.)
Classification of
phase transitions
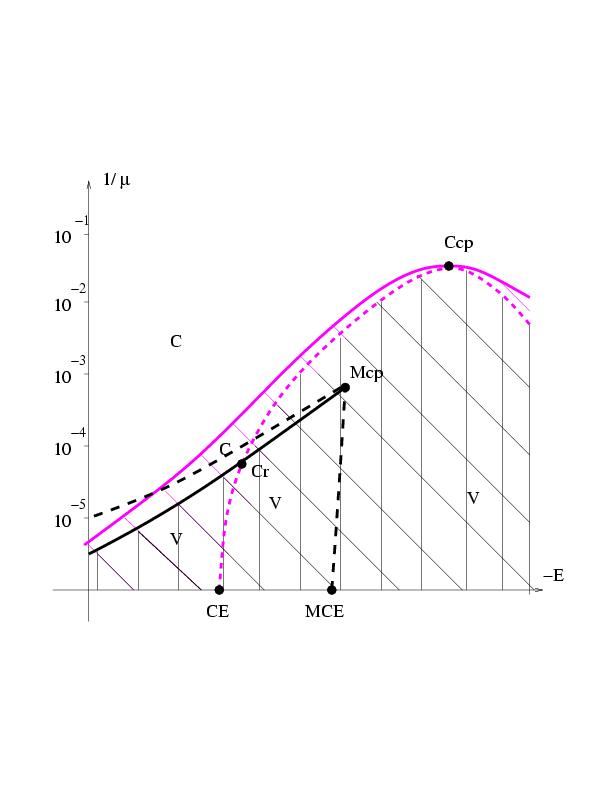
The 2D Euler equations are an example of systems with
long range interactions. Systems with long range
interactions are not additive, which can lead to
inequivalence between the microcanonical and canonical
ensembles. The microcanonical ensemble may show richer
behavior than the canonical one, including negative heat
capacities (the temperature decrease when the energy is
increased) and other non-common behaviors like negative
temperature jumps when the energy is increased, at a
microcanonical critical point.
We have proposed a generalization of Landau classification
for systems with long range interactions that describes
all the possible phase transitions associated with
situations of ensemble inequivalence. The phenomenology
for such phase transitions is richer than the classical
one. We have then predicted new ensemble inequivalence
situations that have never been observed yet and others
than have been observed only after our work
(see Bouchet-Barre-JStatPhys-2005).
Simpler variational
problems for the RSM statistical equilibria
The Robert-Sommeria-Miller equilibrium statistical mechanics predicts the final organization of two dimensional flows. This powerful theory is difficult to handle practically, due to the complexity associated with an infinite number of constraints. Several alternative simpler variational problems, based on Casimirís or stream function functionals, have been considered recently. We have established the relations between all these variational problems, justifying the use of simpler formulations. This provides a drastic mathematical simplifications for the study of equilibria, and increases our physical insight by justifying new physical analogies.
(see Bouchet-PhysicaD-2008).
Previously unobserved phase transitions and ensemble inequivalence for academic ocean models
We propose a theoretical description for the equilibrium states of a large class of models of two-dimensional and geophysical flows. Statistical ensemble inequivalence is found to exist generically in those models, related with the occurrence of peculiar phase transitions in the flow topology. The first examples of a bicritical point in the context of systems with long range interactions is reported. Steady states of academic ocean models, the Fofonoff flows, are studied in the perspective of those results. A more detailed description is proposed in and will be the subject of the forthcoming publication.
(see Venaille-Bouchet-PRL-2009 and Venaille-Bouchet-JStatPhys-2011).
See also related links:
- The 2D-Euler equation is an example of system with long range interaction, please see STATISTICAL MECHANICS OF SYSTEMS WITH LONG RANGE INTERACTIONS