Catégorie : fr

Salambô Dago and Ludovic Bellon, Phys. Rev. Lett. 128, 070604 (2022)
[Article] doi: 10.1103/PhysRevLett.128.070604
[Dataset] doi: 10.5281/zenodo.4807408

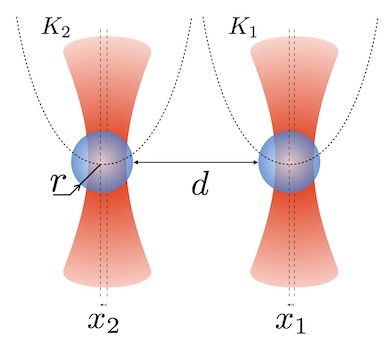
Using a double-well potential as a physical memory, we study with experiments and numerical simulations the energy exchanges during erasure processes, and model quantitatively the cost of fast operation. Within the stochastic thermodynamics framework we find the origins of the overhead to Landauer’s Bound required for fast operations: in the overdamped regime this term mainly comes from the dissipation, while in the underdamped regime it stems from the heating of the memory. Indeed, the system is thermalized with its environment at all time during quasi-static protocols, but for fast ones, the inefficient heat transfer to the thermostat is delayed with respect to the work influx, resulting in a transient temperature rise. The warming, quantitatively described by a comprehensive statistical physics description of the erasure process, is noticeable on both the kinetic and potential energy: they no longer comply with equipartition. The mean work and heat to erase the information therefore increase accordingly. They are both bounded by an effective Landauer’s limit k_BT_effln2, where T_eff is a weighted average of the actual temperature of the memory during the process.

Download pdf
Download data

Basile Pottier and Ludovic Bellon, Journal of Applied Physics 130, 124502 (2021)

[Article] doi:10.1063/5.0060911
[Data set] doi:10.5281/zenodo.5346796

Atomic force microscopy cantilevers are often, intentionally or not, heated at their extremity. We describe a model to compute the resulting temperature field in the cantilever and in the surrounding fluid on a wide temperature range. In air and for common geometries, the heat fluxes in the cantilever and to the environment are of comparable magnitude. We then infer how the fluid–structure interaction is modified due to heating and predict the induced changes in the dynamics of the system. In particular, we describe how the resonance frequencies of the cantilever shift with a temperature increase due to two competing processes: softening of the cantilever and decrease of the fluid inertial effects. Our models are illustrated by experiments on a set of cantilevers spanning the relevant geometries to explore the relative importance of both effects.

Download pdf
Download data

Alex Fontana, Richard Pedurand, Vincent Dolique, Ghaouti Hansali, Ludovic Bellon, Physical Review E 103, 062125 (2021)

[Article] doi: 10.1103/PhysRevE.103.062125
[Data set] doi: 10.5281/zenodo.4696489

 The Fluctuation-Dissipation Theorem (FDT) is a powerful tool to estimate the thermal noise of physical systems in equilibrium. In general however, thermal equilibrium is an approximation, or cannot be assumed at all. A more general formulation of the FDT is then needed to describe the behavior of the fluctuations. In our experiment we study a micro-cantilever brought out-ofequilibrium by a strong heat flux generated by the absorption of the light of a laser. While the base is kept at cryogenic temperatures, the tip is heated up to the melting point, thus creating the highest temperature difference the system can sustain. We independently estimate the temperature profile of the cantilever and its mechanical fluctuations, as well as its dissipation. We then demonstrate how the thermal fluctuations of all the observed degrees of freedom, though increasing with the heat flux, are much lower than what is expected from the average temperature of the system. We interpret these results thanks to a minimal extension of the FDT: this dearth of thermal noise arises from a dissipation shared between clamping losses and distributed damping.

Download pdf
Download data

Basile Pottier and Ludovic Bellon, SciPost Phys. 10, 120 (2021)

[Article] doi: 10.21468/SciPostPhys.10.5.120
[Data set] doi: 10.5281/zenodo.4703793

We report a thermo-optical bistability observed in silicon micro-cantilevers irradiated by a laser beam with mW powers: reflectivity, transmissivity, absorption, and temperature can change by a factor of two between two stable states for the same input power. The temperature dependency of the absorption at the origin of the bistability results from interferences between internal reflections in the cantilever thickness, acting as a lossy Fabry-Pérot cavity. A theoretical model describing the thermo-optical coupling is presented. The experimental results obtained for silicon cantilevers irradiated in vacuum at two different visible wavelengths are in quantitative agreement with the predictions of this model.

Download pdf
Download data

Basile Pottier, Felipe Aguilar, Mickaël Geitner, Francisco Melo, Ludovic Bellon, Journal of Applied Physics 129, 184503 (2021) – Editor’s Pick

[Article] doi: 10.1063/5.0040733
[Data set] doi: 10.5281/zenodo.4629591

When heated, micro-resonators present a shift of their resonance frequencies. We study specifically silicon cantilevers heated locally by laser absorption, and evaluate theoretically and experimentally their temperature profile and its interplay with the mechanical resonances. We include both elasticity and geometry temperature dependency, showing that the latter can account for 20% of the observed shift for the first flexural mode. The temperature profile description takes into account thermal clamping conditions, radiation at high temperature, and lower conductivity than bulk silicon due to phonon confinement. Thanks to a space-power equivalence in the heat equation, scanning the heating point along the cantilever directly reveals the temperature profile. Finally, frequency shift measurement can be used to infer the temperature field with a few percent precision.

Download pdf
Download data

 Salambô Dago, Jorge Pereda, Nicolas Barros, Sergio Ciliberto, and
 Ludovic Bellon, Phys. Rev. Lett. 126, 170601 (2021)

[Article] doi: 10.1103/PhysRevLett.126.170601
[Data set] doi:10.5281/zenodo.4626559

The Landauer principle states that at least k_BT ln 2 of energy is required to erase a 1-bit memory, with k_BT the thermal energy of the system. We study the effects of inertia on this bound using as one-bit memory an underdamped micro-mechanical oscillator confined in a double-well potential created by a feedback loop. The potential barrier is precisely tunable in the few k_BT range. We measure, within the stochastic thermodynamic framework, the work and the heat of the erasure protocol. We demonstrate experimentally and theoretically that, in this underdamped system, the Landauer bound is reached with a 1 % uncertainty, with protocols as short as 100 ms.

Download pdf
Download data

S. Dago, B. Besga , R. Mothe, D. Guéry-Odelin, E. Trizac , A. Petrosyan, L. Bellon, S. Ciliberto, SciPost Phys. 9, 064 (2020)

[Article] doi: 10.21468/SciPostPhys.9.5.064
[Data set] doi:10.5281/zenodo.4242922

We present a detailed theoretical and experimental analysis of Engineered Swift Equilibration (ESE) protocols applied to two hydrodynamically coupled colloids in optical traps. The second particle disturbs slightly (10% at most) the response to an ESE compression applied to a single particle. This effect is quantitatively explained by a model of hydrodynamic coupling. Then we design a coupled ESE protocol for the two particles, allowing the perfect control of one target particle while the second is enslaved to the first. The calibration errors and the limitations of the model are finally discussed in detail.

Download pdf
Download data