Salambô Dago, Sergio Ciliberto, Ludovic Bellon, PNAS 120 e2301742120 (2023)
[Article] doi: 10.1073/pnas.2301742120
[Dataset] doi: 10.5281/zenodo.6572643
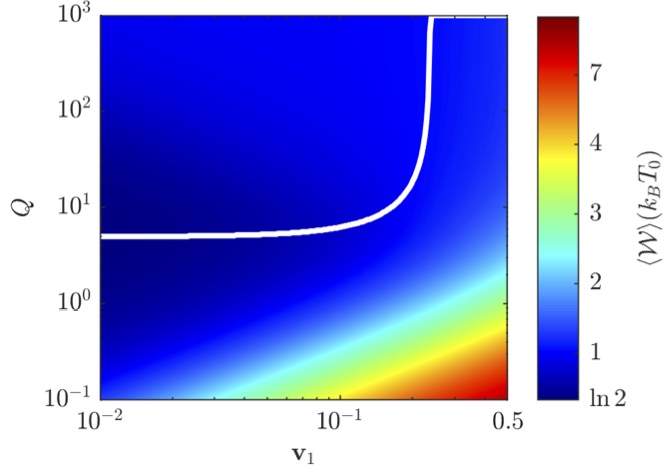
Handling information in the physical world requires energy: Landauer’s principle makes a Landauer’s principle makes a strong connection between information theory and thermodynamics by stating that erasing a one-bit memory at temperature T0 requires an average energy larger than WLB = kBT0 ln2, with kB Boltzmann’s constant. This tiny limit has been saturated in model experiments using quasistatic processes. For faster operations, an overhead proportional to the processing speed and to the memory damping appears. In this article, we show that underdamped systems are a winning strategy to reduce this extra energetic cost. We prove both experimentally and theoretically that, in the limit of vanishing dissipation mechanisms in the memory, the physical system is thermally insulated from its environment during fast erasures, i.e., fast protocols are adiabatic as no heat is exchanged with the bath. Using a fast optimal erasure protocol, we also show that these adiabatic processes produce a maximum adiabatic temperature Ta = 2T0, and that Landauer’s bound for fast erasures in underdamped systems becomes the adiabatic bound: Wa = kBT0.